问题介绍：

自由刚体的定点转动中有一个比较有名的现象：网球拍效应(Tennis Racket Theorem)，又称Dzhanibekov效应。指的是绕转动惯量居中的惯量主轴转动的自由刚体，会发生转动轴翻转的情况，如下:

这种翻转看起来十分反常理：转着转着为什么会翻？还翻得这么快？

下面这个视频演示了绕不同的主轴转动的稳定性的区别：

可以看出绕转动惯量较小的轴和转动惯量较大的轴转动时转动都是稳定的。

学过理论力学的应该都很清楚，如果在刚体坐标架下的角动量空间中看，考查等角动量球和等动能椭球的交线在不同动能大小下的拓扑性质，很容易得到绕不同轴转动时稳定性情况。

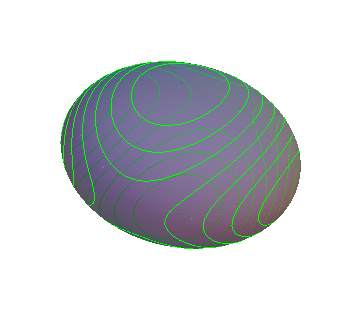
但是，这是做了两次变换后才得到的图像，直观却不直接。有没有什么直接从质点力学的角度看的办法吗？

陶的回答：

在MathOverflow上，陶对别人提出的这个问题给出了一个简单的模型与解释（解释很详细，因此说的很啰嗦），摘录与翻译（翻译的渣求轻拍）如下：

One can see this effect qualitatively from Newtonian first principles (as opposed to Hamiltonian or Lagrangian principles) by looking at a degenerate case, when one moment of inertia is very small and the other two are very close to each other.

这里给出一个能从牛顿力学的观点（而不是从哈密顿或拉格朗日原理）来简单解释的退化的情况：三个主轴转动惯量中有一个很小，而另外两个很接近

More specifically, consider a thin rigid unit disk, initially oriented in the xy plane and centred at the origin (0,0,0). We make the "spherical cow" hypotheses that this disk has infinitesimal thickness and mass, but infinite rigidity. On this disk, we place heavy point masses of equal mass M at the points (1,0,0) and (-1,0,0), and light point masses of equal mass m at the points (0,1,0) and (0,-1,0). Here 0<m<<M, i.e. m should be viewed as negligible with respect to M. (The moments of inertia are then 2m,2M,2(m+M), though we will not explicitly use these moments in the analysis below.) 

具体来说，考虑一个薄的刚体圆盘，初态位于xy平面上，中心位于(0,0,0)。我们使用球形牛假设(*是真空中的球形鸡的梗么...*)，即盘无限薄，无限轻且足够坚硬。在盘上，我们分别将两个质量为M的重质点固着于(1,0,0),(-1,0,0)，两个质量为m的轻质点固着于(0,1,0),(0,-1,0)。这儿有 0<m<<M,即m相对m可以忽略。（在这里质量分别为2m,2M,2(m+M)，在下面的分析中我们不会明确使用这些量）

We now set up the unstable equilibrium by rotating the disk around the y axis. Thus, the light m-masses stay fixed, while the heavy M-masses rotate in the xz plane. This is in equilibrium: there are no net forces on the m-masses, while the rigid disk exerts a centripetal force on the M-masses that keeps them moving in a circular motion on the xz plane. 

我们看一个不稳定的平衡态：盘绕着y轴旋转。这样，轻质点m平衡的停在那里，而重质点M在xz平面旋转。这是一个平衡态：m没有受到净的作用力，而刚体盘给重质点提供向心力使他们绕着xz平面做圆周运动

We can view this equilibrium in rotating coordinates, matching the motion of the M-masses. (Imagine a camera viewing the disk, rotating around the y-axis at exactly the same rate as the disk is rotating.) In this rotating frame, the disk is now stationary (so the m-masses are stuck at (0,±1,0) and the M-masses are stuck at (±1,0,0)), but there is a centrifugal force exerted on all bodies proportional to the distance to the y-axis. The m-masses are on the y-axis and thus experience no centrifugal force; but the M-masses are away from the y-axis and thus experience a centrifugal force, which is then balanced out by the centripetal forces of the rigid disk.

我们可以在转动坐标架中考察这个平衡，即跟上M质点的运动（考虑一个绕着y轴以相同角速度旋转的摄像头正对着圆盘）。在转动系中，圆盘是静态的（因此质点m固着在(0,±1,0)，而质点M固着在(±1,0,0)），但是有一个与到y轴距离成正比的离心力施加在所有物体上。m位于y轴上因此不会受到离心力；但M由于离轴而受到一个被刚体盘的张力平衡掉的离心力。

Now let us perturb the disk a bit, so that the m-masses and M-masses are knocked a little bit out of position (but keeping the centre of mass fixed at (0,0,0)). In particular, the m-masses are knocked away from the y-axis and now experience a little bit of centrifugal force. On the other hand, the rigid disk forces the light m-masses to remain orthogonal to the heavy M-masses, by exerting tension forces between the masses. In the regime where m is negligible compared to M, these tension forces will barely budge the heavy M masses (which therefore remain essentially fixed at (±1,0,0) in the rotating frame), so the effect of these tension forces is to constrain the m-masses to lie in the yz plane (up to negligible errors which we now ignore). Rigidity also keeps the m-masses at a unit distance from the origin, and antipodal to each other, so the m-masses are now constrained to be antipodal points on the unit circle in the yz plane. However, other than this, rigidity imposes no further constraints on the location of the m-masses, which can then move freely as antipodal points in this unit circle.

现在让我们给盘一个微扰，使得M,m都离开平衡位置一点点（但质心仍然保持在(0,0,0)不动）。一方面，轻质点m会由于离开y轴一点点而受到一点离心力。另一方面，刚性圆盘通过张力使得两m间的连线仍然与两M间的连线正交，由于m远小于M，这些张力几乎挪不动两重质点M（因而在转动坐标下M基本维持在(±1,0,0)的位置上），所以这些张力的作用是将m质点限制在yz平面内（最多是有一些可忽略的错误）。刚体也使得质点m到原点的距离始终为1，并使得两质点关于坐标原点对称。所以说两质量为m的质点被限制为yz平面上一个单位圆上的一对对称点。然而，除此之外，刚体没有给两轻质点m的位置加进一步的约束，即它们可以作为单位圆上的对称点自由运动。

The effect of centrifugal force in the rotating frame is now clear: if an m-mass (and its antipode) is perturbed to be a little bit off the y-axis in this unit circle with no initial velocity, then centrifugal force will nudge it a little further off the y-axis, slowly at first but with inexorable acceleration. Eventually it will shoot across the unit circle and then approach the antipode of its previous position. At this point the centrifugal forces act to slow the m-masses down, reversing all the previous acceleration, until one ends up with no velocity at a small distance from the antipode. The process then repeats itself (imagine a marble rolling frictionlessly between two equally tall hills, starting from a position very close to the peak of one of the hills).

在转动坐标架中，离心力的影响现在很明确了：当一个质点m（和它的对称点）受到了一个沿着单位圆偏离y轴的小位置扰动（没有初速度），离心力将会把它进一步拉离y轴，开始很慢但必然会加速。最终它将穿过半个单位圆并到达与其初始位置相对的地方。在这一点离心力将会给质点m减速，抵消之前的加速过程，直到接近初始位置的对称点时速度降为零。这个过程将会不断重复（想象一颗在两座同样高的小山间无摩擦滚动的球，从一个非常接近一座山的山顶的位置出发）。

模拟演示：

这里给出几种情况下陶所说的模型的演示：

绕转动惯量最大的主轴稳定转动

绕转动惯量最小的主轴稳定转动：

翻转运动（在转动系中没有越过平衡点，即陶所说的情形）：

翻转运动（在转动系中越过了平衡点）

另外这里顺便给出陶所说的情形下刚体运动的空间极迹

附绘图的Mma代码如下：

\[Gamma] = 10;(*质量比*)
\[Beta] = -0.01/(\[Gamma] + 1) + 1.01/\[Gamma];(*2E I_1/L^2*)

s = Sqrt[(1 - \[Beta])/\[Gamma]/(\[Gamma] + 1)];
m = (\[Gamma] - 1) (\[Beta] (\[Gamma] + 1) - 1)/(1 - \[Beta]);
\[CapitalOmega]1[t_] := 
Re[Sqrt[(\[Beta] (\[Gamma] + 1) - 1)/\[Gamma]] JacobiCN[s t, m]];
\[CapitalOmega]2[t_] := 
Re[Sqrt[(\[Beta] (\[Gamma] + 1) - 1)/\[Gamma]] JacobiSN[s t, m]];
\[CapitalOmega]3[t_] := 
Re[Sqrt[(1 - \[Beta])/\[Gamma]/(\[Gamma] + 1)] JacobiDN[s t, m]];
\[Theta][t_] := ArcCos[(\[Gamma] + 1) \[CapitalOmega]3[t]];
\[Psi][t_] := Which[
\[CapitalOmega]1[t] >= 0 && \[CapitalOmega]2[t] > 0, 
ArcTan[\[CapitalOmega]1[t]/\[Gamma]/\[CapitalOmega]2[t]],
\[CapitalOmega]1[t] < 0 && \[CapitalOmega]2[t] >= 0, 
2 \[Pi] + ArcTan[\[CapitalOmega]1[t]/\[Gamma]/\[CapitalOmega]2[t]],
\[CapitalOmega]1[t] < 0 && \[CapitalOmega]2[t] < 0, \[Pi] + 
ArcTan[\[CapitalOmega]1[t]/\[Gamma]/\[CapitalOmega]2[t]],
True, \[Pi] + 
ArcTan[\[CapitalOmega]1[t]/\[Gamma]/\[CapitalOmega]2[t]]
];
(*\[Phi][t_]:=NIntegrate[(\[CapitalOmega]1[\[Tau]]^2+\[Gamma] \
\[CapitalOmega]2[\[Tau]]^2)/(\[CapitalOmega]1[\[Tau]]^2+\[Gamma]^2 \
\[CapitalOmega]2[\[Tau]]^2),{\[Tau],0,t}];*)
T = 4 Re[EllipticK[m]]/s; 

(*函数的定义*)

dt = T/64;
tlist = Table[(i - 1/2) dt, {i, 1, 4 T/dt}];
angle = Table[{0, \[Theta][t], \[Psi][t]}, {t, tlist}];
Do[angle[[i, 1]] = angle[[i - 1, 1]] +
dt ((\[CapitalOmega]1[
tlist[[i]]]^2 + \[Gamma] \[CapitalOmega]2[
tlist[[i]]]^2)/(\[CapitalOmega]1[
tlist[[i]]]^2 + \[Gamma]^2 \[CapitalOmega]2[
tlist[[i]]]^2) + (\[CapitalOmega]1[
tlist[[i - 1]]]^2 + \[Gamma] \[CapitalOmega]2[
tlist[[i - 1]]]^2)/(\[CapitalOmega]1[
tlist[[i - 1]]]^2 + \[Gamma]^2 \[CapitalOmega]2[
tlist[[i - 1]]]^2))
, {i, 2, Length[angle]}]

(*数据的计算*)

file = Table[
Graphics3D[{GeometricTransformation[{
Gray, Cylinder[{{0, 0, -0.02}, {0, 0, 0.02}}, 1],
Red, Sphere[{1, 0, 0}, 0.15], Sphere[{-1, 0, 0}, 0.15],
Green, Sphere[{0, 1, 0}, 0.05], Sphere[{0, -1, 0}, 0.05],
Blue, {Arrow[{{-1.15, 0, 0}, {1.15, 0, 0}}], 
Arrow[{{0, -1.15, 0}, {0, 1.15, 0}}], 
Arrow[{{0, 0, -1.15}, {0, 0, 1.15}}]}
}, {RotationMatrix[-angle[[i, 1]], {0, 0, 
1}].RotationMatrix[-angle[[i, 2]], {1, 0, 
0}].RotationMatrix[-angle[[i, 3]], {0, 0, 1}], {0, 0, 
0}}], {Line[{{-1.15, 0, 0}, {1.15, 0, 0}}], 
Line[{{0, -1.15, 0}, {0, 1.15, 0}}], 
Line[{{0, 0, -1.15}, {0, 0, 1.15}}]}}
, Boxed -> False, ImageSize -> 500]
, {i, 1, Length[angle]}];
ListAnimate[file, AnimationRate -> 8]

(*动画生成*)